

That is (restricting our attention to periodic complex exponentials), if e j k t is an input to the LTI system, then the output is H ( j k) e j k t, where H ( j k) is the frequency response of the systsem evaluated at k. But if the system is LTI to begin with, would their be transients in the output if a sinusoidal signal, or complex exponential, is applied? It can be shown mathematically that the output of an LTI system when a complex exponential is applied is just a scaled version of the input, with no transient terms. All LTI systems possess the eigenfunction property for complex exponential inputs. Why is it that when we talk about systems, specifically systems with dynamics described by differential equations, we speak of a “steady-state” sinusoidal output? This seems to imply that transients are always present.
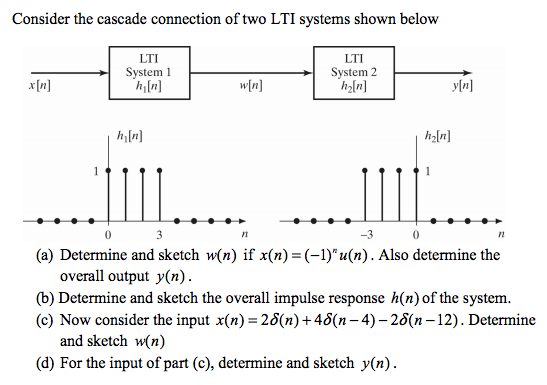
When a linear constant coefficient differential equation with zero initial conditions can represent a causal LTI system.
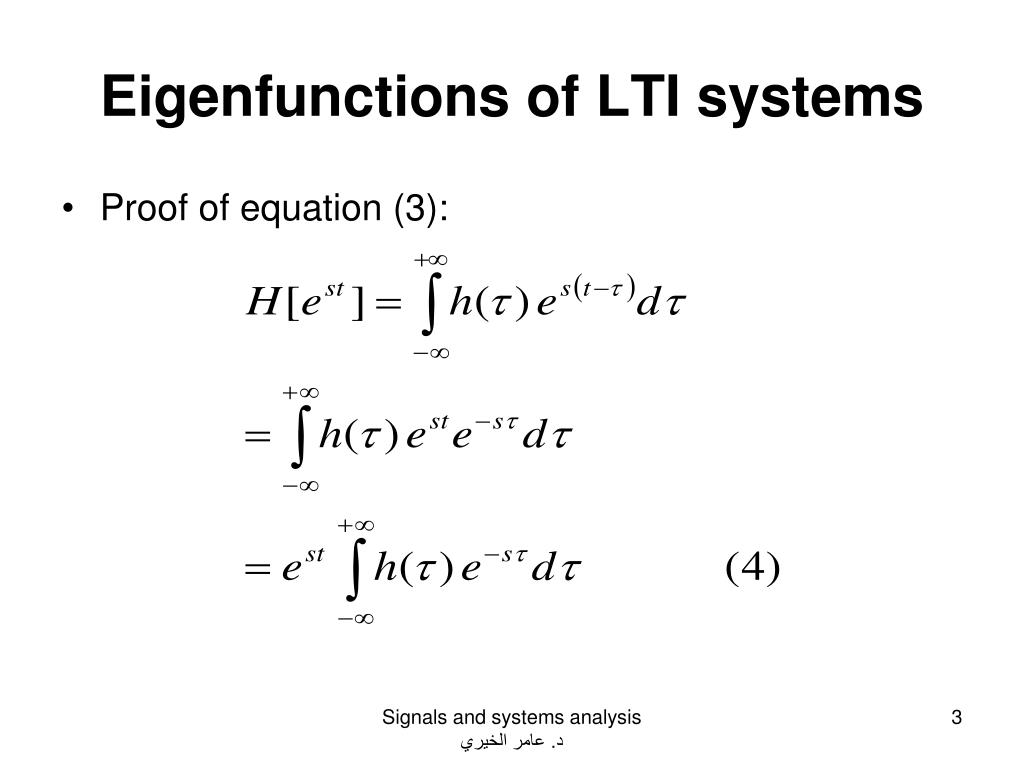
That is (restricting our attention to periodic complex exponentials), if $e^$, where $H(jomega_k)$ is the frequency response of the systsem evaluated at $omega_k$. All LTI systems possess the eigenfunction property for complex exponential inputs.


 0 kommentar(er)
0 kommentar(er)
